Crucial Instances in the Principia
Kirsten Walsh writes…
In the General Scholium, which concluded later editions of Principia, Newton described the work as ‘experimental philosophy’:
In this experimental philosophy, propositions are deduced from phenomena and are made general by induction. The impenetrability, mobility, and impetus of bodies, and the laws of motion and the law of gravity have been found by this method.
On this blog, I have argued that we should take this statement at face value. In support, I have emphasised similarities between Newton’s work in optics and mechanics. For example, I have considered the kind of evidence provided in each work, arguing that both the Principia’s ‘phenomena’ and the Opticks’s ‘experiments’ are idealisations based on observation, and that they perform the same function: isolating explananda. I have also emphasised Newton’s preoccupation in the Principia with establishing his principles empirically. Finally, I have suggested that this concern with experimental philosophy, in combination with his use of mathematics, made Newton’s method unique.
In my last blog post, I wondered if we should regard Newton’s methodology as an extension of the Baconian experimental method, or as something more unique. I have written many blog posts discussing the Baconian aspects of Newton’s optical work (for example, here, here and here), but the Baconian aspects of the Principia are less well-established. I can identify at least three possible candidates for Baconianism in the Principia. The first, suggested by Daniel Schwartz in recent conversation, is that book 3 contains what might be interpreted as Baconian ‘crucial instances’. The second, discussed by Steffen Ducheyne, is that Newton’s argument for universal gravitation resembles Bacon’s method of induction. The third, discussed by Mary Domski, is that the mathematical method employed in the Principia should be viewed as part of the seventeenth-century Baconian tradition. In this post, I’ll focus on Schwartz’s suggestion—the possibility there is a crucial instance in book 3 of the Principia—I’ll address the rest in future posts.
To begin, what is a ‘crucial instance’? For Bacon, crucial instances (instantiae crucis) were a subset of ‘instances with special powers’ (ISPs). When constructing a Baconian natural history, ISPs were experiments, procedures, and instruments that were held to be particularly informative or illuminative of aspects of the inquiry. These served a variety of purposes. Some functioned as ‘core experiments’, introduced at the very beginning of a natural history, and serving as the basis for further experiments. Others played a role later in the process. This included experiments that were supposed to be especially representative of a certain class of experiments, tools and experimental procedures that provided interesting investigative shortcuts, and model examples that came close to providing theoretical generalisations.
Crucial instances are part of a subset of ISPs that were supposed to aid the intellect by “warning against false forms or causes”. When two possible explanations seemed equally good, then the crucial instance was employed to decide between them. To this end, it performed two functions: the negative function was to eliminate all possible explanations except the correct one; the positive function was to affirm the correct explanation.
According to Claudia Dumitru, Bacon’s crucial instances have a clear structure:
- Specify the explanandum;
- Consider the competing explanations (these are assumed to exhaust the possibilities);
- Derive a consequence from one explanation that is incompatible with the other explanation(s);
- Test that consequence.
Are there any arguments in the Principia that look like crucial instances? I think there’s at least one: Newton’s famous ‘Moon test’. Let’s have a look at it.
In proposition 4 book 3, Newton used his Moon test to establish that “The moon gravitates toward the earth and by the force of gravity is always drawn back from rectilinear motion and kept in its orbit”. Here, Newton argued that the inverse-square centripetal force, keeping the moon in orbit around the Earth, is the same force that, say, makes an apple fall to the ground, namely, gravity. I think we can tease out the features of a Baconian crucial instance from Newton’s reasoning here.
Firstly, there is an explanandum: what kind of force keeps the Moon in its orbit and prevents it from flying off into space? Secondly, two possible explanations are provided: the force is either (a) the same force that that acts on terrestrial objects, namely, gravity; or (b) a different force. Thirdly, we have a consequence of (a) that is incompatible with (b): if the moon were deprived of rectilinear motion, and allowed to fall towards Earth, it would begin falling at the rate of 15 1/12 Paris feet in the space of one minute, accelerating so that at the Earth’s surface it would fall 15 1/12 Paris feet in a second. Finally, we see a test of that consequence: the calculations based on the size and motion of the Moon, and its distance from the Earth. The results are taken to support (a) and refute (b).
I have three concluding remarks to make.
Firstly, interpreting the Moon test as a crucial instance involves ‘rational reconstruction’. In the text, Newton starts by calculating the rate at which the Moon would fall, and shows that this supports proposition 4. But I think my reading of this as a crucial instance is supported by Newton’s concluding remarks:
For if gravity were different from this force, then bodies making for the earth by both forces acting together would descend twice as fast, and in the space of one second would by falling describe 301/6 Paris feet, entirely contrary to experience.
Here, Newton described the Moon test as a crucial instance: he used an observation to choose between two competing explanations of the explanandum.
Secondly, when looking for crucial instances in the Principia, it might be tempting to start with the phenomena, listed at the beginning of book 3. Elsewhere, I have argued that these resemble Newton’s experiments in the Opticks, which function as instances with special powers. But the label ‘crucial instance’ describes the function, not the content, of an empirical claim. And so, to see if they provide crucial instances, we need to consider how the phenomena are used. In fact, I think they do provide crucial instances for Newton’s rejection of Cartesian vortex theory in favour of universal gravitation, found at the end of book 2. But again, this requires rational reconstruction.
Finally, there is the issue of historical influence. I have shown that Newton employed the Moon test to decide between two competing explanations, and that this argument resembles one of Bacon’s crucial instances. However, one might think that this was simply a good approach to empirical support, and that Newton was using his common-sense. So perhaps we shouldn’t take this to indicate (direct or indirect) influence. And so I have a question for our readers: was this style of reasoning uniquely Baconian?
Newton the empiricist?
Kirsten Walsh writes…
Recently, Zvi Biener and Eric Schliesser’s long-awaited volume, Newton and Empiricism, appeared on the shelves. The book is an excellent collection of papers, which makes a significant new contribution to the field. Today I want to focus on one aspect of this volume: the decision to frame the collection in terms of empiricism rather than experimental philosophy.
Over the last four years, we have provided many arguments for the superiority of the ESD over the RED. An important line of argument has been to show that ‘experimental philosophy’ and ‘speculative philosophy’ were the key terms of reference used by the actors themselves, and that they characterised their own work in terms of this division. For example, I have argued here, here, here and here that Newton is best understood as an experimental philosopher.
In their introduction, Biener and Schliesser explain their decision. They acknowledge the ‘Otago School’, and argue that, while in general there may be some good reasons to prefer the ESD to the RED, they see various problems with labelling Newton an ‘experimental philosopher’. Their concerns amount to the following: labelling Newton an ‘experimental philosopher’ obscures the idiosyncrasies of his approach to natural philosophy. They argue, firstly, that the label belies the significant influence of non-experimental philosophers on Newton’s methodology, for example those who influenced his mathematical focus. Secondly, that the label unhelpfully groups Newton with Boyle and Locke, when many features of his work support a different grouping. For example, Newton’s mathematical-system building suggests that his work should be grouped with Descartes’. Thirdly, they argue that the fact that Newton did not employ the label himself until after the publication of the first edition of the Principia suggests that he did not fully identify with the label.
These are important issues about the ESD and Newton’s place in it. So today I want to reflect on the broad problem of Newton’s idiosyncratic position. I argue that Newton’s divergence from Baconian tradition of the Royal Society is best seen as a development of experimental philosophy.
On this blog, I have sketched many features of Newton’s natural philosophical methodology. I have argued that, if we look at Newton from within the framework of the ESD, he can be neatly and easily identified as an experimental philosopher. His use of queries, his cautious approach to hypotheses, and his many methodological statements decrying the construction of metaphysical systems, suggest that this is a label that Newton would have been comfortable with. However, there is an important caveat to note: while Newton was clearly influenced by the Baconian experimental tradition, he did not consider himself a Baconian experimental philosopher.
In the earliest statements of his mathematico-experimental approach, Newton set up his position in opposition to the Baconian experimental philosophers. In these passages, one feature of Newton’s methodology stands out in explicit rejection of the Baconian method: his claims to certainty. This feature, in itself, is not very significant – many experimental philosophers believed that, in the end, natural philosophy would be a form of scientia, i.e. a system of knowledge demonstrated from certain axioms. Indeed, Bacon shared this ideal of certainty. He thought that his method of induction could get around the problems usually associated with ampliative inference and deliver knowledge of the essences of things. Thus, Bacon’s method of natural history was ultimately supposed to provide the axioms on which scientia could be founded. The challenge, which everyone agreed on, was to discover those axioms on which the system would be built.
Newton and the Baconians seem to diverge on their responses to this challenge. Baconian experimental philosophers recommended that one should have all the facts before formulating generalisations or theories. In contrast, Newton thought that a few, or even just one, well-constructed experiment might be enough – provided you used it in the right way. This shows that Newton took a different view of the role of evidence in natural philosophy. This divergence amounts to three key differences between Newton and the Baconian experimental philosophers:
- Where the Baconian experimental philosophers advocated a two-stage model, in which construction of natural histories preceded theory construction, Newton appeared to reject this two-stage approach. Newton commenced theory-building before his knowledge of the facts was complete.
- Related to (1), the Baconian experimental philosophers conceived of phenomena as immediate facts, acquired via observation, and hence pre-theoretic. In contrast, Newton’s phenomena were generalised regularities, acquired via mediation between observation and theory.
- For the Baconian experimental philosophers, queries were used to give direction and define the scope of the inquiry. But Newton’s queries were more focussed on individual experiments.
There is strong textual evidence that the ESD was operative in Newton’s early natural philosophical work. We have good reason to suppose that Newton regarded his natural philosophical pursuits as experimental philosophy. This becomes clearer in Newton’s later work. For instance, in the General Scholium to the Principia (1713), Newton explicitly described his work as ‘experimental philosophy’ – indeed, Peter Anstey has noted that Roger Cotes also recognised this feature of Newton’s work. We also have good reason to suppose that, in important ways, Newton saw his work as aligned with the Royal Society and, by extension, with the Baconian movement. But Newton was also a mathematician, and he saw a role for mathematical reasoning in experimental philosophy. In many ways, it was this mathematical approach that led to his divergence from the Baconian experimental philosophy.
Biener and Schliesser are right to draw attention to the ways in which Newton’s position diverged from the experimental tradition of the Royal Society. However, they fail to recognise that Newton’s position diverged in a way that should be viewed as a development of this tradition. Indeed, the ‘Newtonian experimental philosophy’ eventually replaced the experimental philosophy of Boyle, Hooke and the other early members of the Royal Society. The label ’empiricism’ has no such historical relevance. But, more on this another time…
‘Epistemic amplification’ and Newton’s laws
Kirsten Walsh writes…
In my last post, I considered the experimental support Newton offers for his laws of motion. In the scholium to the laws, Newton argues that his laws of motion are certainly true. However, in support he only cites a handful of experiments and the agreement of other mathematicians. I suggested that the experiments discussed do support his laws, but only in limited cases. This justifies their application in Newton’s mathematical theory, but does not justify Newton’s claims to certainty. In this post, I will speculate that the laws of motion were in fact better established than Newton’s discussion suggests. I introduce the notion ‘epistemic amplification’ – suggesting that Newton’s laws gain epistemic status by virtue of their relationship to the propositions they entail. That is, by reasoning mathematically from axioms to theorems, the axioms obtained higher epistemic status, and so the reasoning process effectively amplified the epistemic status of the axioms.
I am not arguing that epistemic amplification captures Newton’s thinking. In fact, Newton explicitly stated that epistemic gain was not possible. For him, the best one could achieve was avoiding epistemic loss. (I have discussed Newton’s aims of certainty and avoiding epistemic loss here and here.) I suggest that, objectively speaking, the epistemic status of Newton’s laws increases over the course of the Principia.
To begin, recall that Newton has two projects in relation to the laws:
- The specification of the laws as the axioms of a mathematical system; and
- The justification of laws as first principles in natural philosophy.
Let’s consider the first project. In addition to the support of mathematicians and the experiments that Newton cites, it is plausible that the epistemic status of the laws increases by virtue of their success in the mathematical system: in particular, by entailing Keplerian motion. Kepler’s rules and Newton’s laws of motion have independent evidence: as we have seen, Newton’s laws are weakly established by localised experiments and the ‘agreement of mathematicians’; Kepler’s rules are established by observed planetary motion and were widely accepted by astronomers prior to the Principia. Newton’s laws entail Kepler’s rules, which boosts Newton’s justification for his laws. Moreover, Newton’s laws provide additional support for Kepler’s rules, by telling us about the forces required to produce such motions. The likelihood of the two theories is coupled: evidence for one carries over to the other. So Newton’s laws also boost the justification for Kepler’s rules. Thus, Newton achieves epistemic gain: the epistemic status of the laws, qua mathematical axioms, has increased by virtue of their relationship to Kepler’s rules.
Now let’s consider the second project – the application of the laws to natural philosophy. Again, the discussion in the scholium justifies their use, but not their certainty. I now suggest that these laws, as physical principles, gain epistemic status through confirmation of Newton’s theory. This occurs in book 3, when Newton explicitly applies his mathematical theory to natural phenomena. As I have previously discussed, the phenomena (i.e. the motions of the planets and their moons) are employed as premises in Newton’s argument for universal gravitation. However, the phenomena also support the application of the mathematical theory to the physical world: they show that the planets and their moons move in ways that approximate Keplerian motion. As we saw above, the laws of motion entail Kepler’s rules. So, since the phenomena support Kepler’s rules, they also support the laws of motion. So this is a straightforward case of theory-confirmation.
There is also scope for theory-testing in book 1. Each time Newton introduces a new factor (e.g. an extra body, or a resisting medium), the mathematical theory is tested. For instance, the contrasting versions of the harmonic rule in one-body and two-body model systems provides a test: it allows the phenomena to empirically decide between two theories, one involving singly-directed central forces, the other involving mutually-interactive central forces. Similarly, the contrasting two-body and three-body mathematical systems provide a test: they allow the phenomena to select between a theory involving pair-wise interactions and a theory involving universal mutual interaction. Moreover, in the final section of book 2, Newton shows that, unlike his theory, Cartesian vortex theory does not predict Keplerian motion. Thus, the phenomena seem to support his theory, and by extension the laws of motion, and to refute the theory of vortices. Again, the laws seem to gain support by virtue of their relationship to the propositions they entail.
To summarise, Newton claims that his laws are certainly true, but the support he gives is insufficient. Here, I have sketched an account in which Newton’s laws gain epistemic status by virtue of their relationship to the propositions they entail. ‘Epistemic amplification’ is certainly not something which Newton himself would have had truck with, but the term does seem to capture the support actually acquired by Newton’s laws in the Principia. What do you think?
Are Newton’s Laws Experimentally Confirmed?
Kirsten Walsh writes…
Previously on this blog, I have argued that the combination of mathematics, experiment and certainty are an enduring feature of Newton’s methodology. I have also highlighted the epistemic tension between experiment and mathematical certainty found in Newton’s work. Today I shall examine this in relation to Newton’s ‘axioms or laws of motion’.
In the scholium to the laws, Newton argues that his laws of motion are certainly true. In support, however, he cites a handful of experiments and the agreement of other mathematicians: surprisingly weak justification for such strong claims! In this post, I show how Newton’s appeals to experiment justify the axioms’ inclusion in his system, but not with the certainty he claims.
Newton begins:
- “The principles I have set forth are accepted by mathematicians and confirmed by experiments of many kinds.”
Newton expands on this claim, discussing firstly, Galileo’s work on the descent of heavy bodies and the motion of projectiles, and secondly, the work conducted by Wren, Wallis and Huygens on the rules of collision and reflection of bodies. He argues that:
- The laws and their corollaries have been accepted by mathematicians such as Galileo, Wren, Wallis and Huygens (the latter three were “easily the foremost geometers of the previous generation”);
- The laws and their corollaries have been invoked to establish several theories involving the motions of bodies; and
- The theories established in (2) have been confirmed by the experiments of Galileo and Wren (which, in turn confirms the truth of the laws).
These claims show us that Newton regards his laws as well-established empirical propositions. However, Newton recognises that the experiments alone are not sufficient to establish the truth of the laws. After all, the theories apply exactly only in ideal situations, i.e. situations involving perfectly hard bodies in a vacuum. So Newton describes supplementary experiments that demonstrate that, once we control for air resistance and degree of elasticity, the rules for collisions hold. He concludes:
- “And in this manner the third law of motion – insofar as it relates to impacts and reflections – is proved by this theory [i.e. the rules of collisions], which plainly agrees with experiments.”
This passage suggests that the rules of collisions support a limited version of law 3, “to any action there is always an opposite and equal reaction”, and that the rules themselves appear to hold under experimental conditions. However, this doesn’t show that law 3 is universal: which Newton needs to establish universal gravitation. This argument is made by showing how the principle may be extended to other cases.
Firstly, Newton extends law 3 to cases of attraction. He considers a thought experiment in which two bodies attract one another to different degrees. Newton argues that if law 3 does not hold between these bodies the system will constantly accelerate without any external cause, in violation of law 1, which is a statement of the principle of inertia. Therefore, law 3 must hold. As the principle of inertia was already accepted, this supports the application of law 3 to attraction.
Newton then demonstrates law 3’s application to various machines. For example, he argues that two bodies suspended from opposite ends of a balance have equal downward force if their respective weights are inversely proportional to the distances between the axis of the balance and the points at which they are suspended. And he argues that a body, suspended on a pulley, is held in place by a downward force which is equal to the downward force exerted by the body. Newton explains that:
- “By these examples I wished only to show the wide range and the certainty of the third law of motion.”
What these examples in fact show is the explanatory power of the laws of motion – particularly law 3 – in natural philosophy. Starting with collision, which everyone accepts, Newton expands on his cases to show how law 3 explains many different physical situations. Why wouldn’t a magnet and an iron floating side-by-side float off together at an increasing speed? Because, by law 3, as the magnet attracts the iron, so the iron attracts the magnet, causing them to press against one another. Why do weights on a balance sometimes achieve equilibrium? Because, by law 3, the downward force at one end of the balance is equal to the upward force at the other end of the balance. These examples demonstrate law 3’s explanatory breadth. But these examples do not give us a compelling reason to think that law 3 should be extended to gravitational attraction (which seems to require some kind of action, or attraction, at a distance).
Newton, clearly, is convinced of the strength of his laws of motion. But this informal, discussion of the experiments he appeals to shows that he ought not be so convinced. As I see it, Newton has two projects in relation to his laws:
1) The specification of the laws as the axioms of a mathematical system; and
2) The justification of laws as first principles in natural philosophy.
I suggest that the experiments discussed give strong support for the laws in limited cases. This justifies their application in Newton’s mathematical model, but it does not justify Newton’s claims to certainty. In modern Bayesian terms, we might say that Newton’s laws have high subjective priors. In my next post, I shall sketch an account in which Newton’s laws gain epistemic status by virtue of their relationship to the propositions they entail.
Understanding Newton’s Experiments as Instances of Special Power
Kirsten Walsh writes…
In my last few posts, I have been discussing the nature of observations and experiments in Newton’s Opticks. In my first post on this topic, I argued that Newton’s distinction between observation and experiment turns on their function. That is, the experiments introduced in book 1 offered individual, and crucial, support for particular propositions, whereas the observations introduced in books 2 and 3 only supported propositions collectively. In my next post, I discussed the observations in more detail, arguing that they resemble Bacon’s ‘experientia literata’, the method by which natural histories were supposed to be generated. At the end of that post, I suggested that, in contrast to the observations, Newton’s experiments look like Bacon’s ‘instances of special power’, which are particularly illuminating cases introduced to provide support for specific propositions. Today I’ll develop this idea.
Note, before we continue, that there are two issues here that can be treated independently of one another. One is establishing the extent of Bacon’s historical influence on Newton; the other is establishing the extent to which Bacon’s methodology can illuminate Newton’s. In this post I am doing the latter – using Bacon’s view only as an interpretive tool.
Identifying ‘instances of special power’ (ISPs) was an important step in the construction of a Baconian natural history. ISPs were experiments, procedures, and instruments that were held to be particularly informative or illuminative. These served a variety of purposes. Some functioned as ‘core experiments’, introduced at the very beginning of a natural history, and serving as the basis for further experiments. Others played a role later in the process. They included experiments that were supposed to be especially representative of a certain class of experiments, tools and experimental procedures that provided interesting shortcuts in the investigation, and model examples that came very close to providing theoretical generalisations. In some cases, a collection of ISPs constituted a natural history.
The following features were typical of ISPs. Firstly, they were considered to be particularly illuminating experiments, procedures or tools. For example, a crucial instance, or a particularly clear or informative experiment, or experimental procedure. Secondly, they were supposed to be replicated. On Bacon’s view, replication was not merely an exercise for verifying evidence; it was an exercise for the mind, ensuring that one had truly grasped the phenomenon. Thirdly, they were versatile, in that they could be used in several different ways. As we shall see, the experiments of book 1 display these essential features.
In book 1 of the Opticks, Newton employed a method of ‘proof by experiments’ to support his propositions. Each experiment was introduced to reveal a specific property of light, which in turn proved a particular proposition. We know that Newton conducted many experiments in his optical investigations, so why did he present the experiments as he did, when he did? When we consider Newton’s experiments alongside Bacon’s instances of special power, common features start to emerge.
Firstly, for each proposition he asserted, Newton introduced a small selection of experiments in support – those that he considered to be particularly illuminating or, in his own words, “necessary to the Argument”. Unlike in his first paper, in the Opticks, Newton did not label any experiments ‘experimentum crucis’. But his use of terms such as ‘necessary’ and ‘proof’ make it clear that these experiments were supposed to provide strong support: just like ISPs.
Secondly, Newton usually provided more than one experiment to support each proposition. These were listed in order of increasing complexity and were carefully described and illustrated. That Newton took this approach, as opposed to just reporting on their results, suggests that these experiments were supposed to be an exercise for the reader: they were about more than just proof or confirmation of the proposition. The reader was supposed either to be able to replicate the experiment, or at least to understand its replicability. Starting with the simplest experiment, Newton led his reader by the hand through the relevant properties of light, to ensure that they were properly grasped. Like Bacon’s ISPs, then, Newton’s experiments were intended to be replicated.
Thirdly, Newton’s experiments were recycled in a variety of roles in the Opticks. For example, the experiments he used to support proposition 2 part II were experiments 12 and 14 from part I. Newton introduced and developed these experiments in several different contexts to illuminate and support different propositions. Again, this is typical of Bacon’s ISPs.
And so, Newton’s experiments in the Opticks play a role analogous to Bacon’s instances of special power, and thinking of them as such explains why they are presented as they are. They are particularly illuminating cases that are introduced to provide support for specific propositions. Newton selected the experiments which best functioned as ISPs for inclusion in the Opticks. Moreover, seen in this light, the seemingly disparate set of experiments start to look like a far more cohesive collection, or a natural history.
Many commentators have emphasised the ways that Newton deviated from Baconian method. Through this sequence of posts, I have argued that the Opticks provides a striking example of conformity to the Baconian method of natural history.
Workshop: Mathematics and methodology from Newton to Euler
University of Sydney
20 March, 2014
9:15-5:30
Program:
- 9.15 Katherine Dunlop (Texas): ‘Christian Wolff on Newtonianism and Exact Science’
- 10.45 Coffee
- 11.00 Peter Anstey (Sydney): ‘From scientific syllogisms to mathematical certainty’
- 12.30 Lunch
- 2.00 Kirsten Walsh (Otago): ‘Newton’s method’
- 3.30 Stephen Gaukroger (Sydney): ‘D’Alembert, Euler and mid-18th century rational mechanics: what mechanics does not tell us about the world’
- 5.00 Wind up
Location: Common Room 822, Level 8, Brennan MacCallum Building
Contact: Prof Peter Anstey
Phone: 61 2 9351 2477
Email: peter.anstey@sydney.edu.au
RSVP: Here
Observation and Experiment in the Opticks: A Baconian Interpretation
Kirsten Walsh writes…
In a recent post, I considered Newton’s use of observation and experiment in the Opticks. I suggested that there is a functional (rather than semantic) difference between Newton’s ‘experiments’ and ‘observations’. Although both observations and experiments were reports of observations involving intervention on target systems and manipulation of independent variables, experiments offered individual, and crucial, support for particular propositions, whereas observations only supported propositions collectively.
At the end of the post, I suggested that, if we view them as complex, open ended series’ of experiments, the observations of books 2 and 3 look a lot like what Bacon called ‘experientia literata’, the method by which natural histories were supposed to be generated. In this post, I’ll discuss this suggestion in more detail, following Dana Jalobeanu’s recent work on Bacon’s Latin natural histories and the art of ‘experientia literata’.
The ‘Latin natural histories’ were Bacon’s works of natural history, as opposed to his works about natural history. A notable feature of Bacon’s Latin natural histories is that they were produced from relatively few ‘core experiments’. By varying these core experiments, Bacon generated new cases, observations and facts. The method by which this generation occurs is called the art of ‘experientia literata’. Experientia literata (often referred to as ‘learned experience’) was a late addition to Bacon’s program, developed in De Augmentis scientiarum (1623). It is a tool or technique for guiding the intellect. By following this method, discoveries will be made, not by chance, but by moving from one experiment to the next in a guided, systematic way.
The following features were typical of the experientia literata:
- The series of observations was built around a few core experiments;
- New observations were generated by the systematic variation of experimental parameters;
- The variation could continue indefinitely, so the observation sequence was open-ended;
- The experimental process itself could reveal things about the phenomena, beyond what was revealed by a collection of facts;
- The trajectory of the experimental series was towards increasingly general facts about the phenomena; and
- The results of the observations were collated and presented as tables. These constituted the ‘experimental facts’ to be explained.
Now let’s turn to Newton’s observations. For the sake of brevity, my discussion will focus on the observations in book 2 part I of the Opticks, but most of these features are also found in the observations of book 2 part IV, and in book 3 part I.
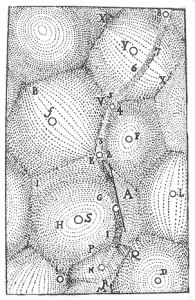
Figure 1 (Opticks, book 2 part I)
The Opticks book 2 concerned the phenomenon now known as ‘Newton’s Rings’: the coloured rings produced by a thin film of air or water compressed between two glasses. Part I consisted of twenty-four observations. Observation 1 was relatively simple: Newton pressed together two prisms, and noticed that, at the point where the two prisms touched, there was a transparent spot. The next couple of observations were variations on that first one: Newton rotated the prisms and noticed that coloured rings became visible when the incident rays hit the prisms at a particular angle. Newton progressed, step-by-step, from prisms to convex lenses, and then to bubbles and thin plates of glass. He varied the amount, colour and angle of the incident light, and the angle of observation. The result was a detailed, but open ended, survey of the phenomena. Part II consisted of tables that contained the results of part I. These constituted the experimental facts to be explained in propositions in part III. In part IV, Newton described a new set of observations, which built on the discussions of propositions from part III.
When we consider Newton’s observations alongside Bacon’s experientia literata, we notice some common features.
Firstly, the series of observations was built around the core experiment involving pressing together two prisms to observe the rings that appeared.
Secondly, new observations were generated by the variation of experimental parameters: i.e. new observations were generated, first by varying the obliquity of the incident rays, then by varying the glass instruments, then by varying the colour of the incident light, and so on.
Thirdly, the sequence of observations was open-ended. Newton could have extended the sequence by varying the medium, or some other experimental parameter. Moreover, at the end of the sequence, Newton noted further variations to be carried out by others, which might yield new or more precise observations.
Fourthly, the experimental process itself revealed things about the phenomenon, beyond what was revealed by a collection of facts. For example, in observation 1, Newton noticed that increasing the pressure on the two prisms produced a transparent spot. The process of varying the pressure, and hence the thickness of the film of air between the two prisms, suggested to Newton a way of learning more about the phenomenon of thin plates. He realised he could quantify the phenomenon by introducing regularly curved object glasses, which would make the variation in thickness regular, and hence, calculable.
Fifthly, the trajectory of the experimental series was towards increasingly general facts about the phenomenon. Newton began by simply counting the number of rings and describing the sequence of colours under specific experimental parameters. But eventually he showed that the number of rings and their colours was a function of the thickness and density of the film. Thus, he was able to give a much broader account of the phenomenon.
Finally, these general results were collated and presented as tables in part II. Thus, the tables in part II constituted the facts to be explained by propositions in part III.
Many commentators have emphasised the ways that Newton deviated from Baconian method. However, when viewed in this light, book 2 of the Opticks provides a striking example of conformity to the Baconian method of natural history: Newton led the reader from observations in part I, to tables of facts in part II, to propositions in part III. Moreover, it ended with a further series of observations in part IV, emphasising the open-endedness of the art of experientia literata.
In contrast to the observations in book 2, Newton’s experiments in book 1 look like Bacon’s ‘instances of special power’, which are particularly illuminating cases introduced to provide support for specific propositions. I’ll discuss this next time. For now, I’d like to hear what our readers think of my Baconian interpretation of Newton’s observations.
Observation, experiment and intervention in Newton’s Opticks
Kirsten Walsh writes…
In my last post, my analysis of the phenomena in Principia revealed a continuity in Newton’s methodology. I said:
- In the Opticks, Newton isolated his explanatory targets by making observations under controlled, experimental conditions. In Principia, Newton isolated his explanatory targets mathematically: from astronomical data, he calculated the motions of bodies with respect to a central focus. Viewed in this way, Newton’s phenomena and experiments are different ways of achieving the same thing: isolating explananda.
In this post, I’ll have a closer look at Newton’s method of isolating explananda in the Opticks. It looks like Newton made a distinction between experiment and observation: book 1, contained ‘experiments’, but books 2 and 3, contained ‘observations’. I’ll argue that the distinction in operation here was not the standard one, which turns on level of intervention.
In current philosophy of science, the distinction between experiment and observation concerns the level of intervention involved. In scientific investigation, intervention has two related functions: isolating a target system, and creating novel scenarios. On this view, experiment involves intervention on a target system, and manipulation of independent variables. In contrast, the term ‘observation’ is usually applied to any empirical investigation that does not involve intervention or manipulation. This distinction is fuzzy at best: usually level of intervention is seen as a continuum, with observation nearer to one end and experiment nearer to the other.
If Newton was working with this sort of distinction, then we should find that the experiments in book 1 involve a higher level of intervention than the observations in books 2 and 3. That is, in contrast to the experiments in book 1, the observations should involve fewer prisms, lenses, isolated light rays, and artificially created scenarios. However, this is not what we find. Instead we find that, in every book of the Opticks, Newton employed instruments to create novel scenarios that allowed him to isolate and identify certain properties of light. It is difficult to quantify the level of intervention involved, but it seems safe to conclude that Newton’s use of the terms ‘observation’ and ‘experiment’ doesn’t reflect this distinction.
To understand what kind of distinction Newton was making, we need to look at the experiments and observations more closely. In Opticks book 1, Newton employed a method of ‘proof by experiments’ to support his propositions. Each experiment was designed to reveal a specific property of light. Consider for example, proposition 1, part I: Lights which differ in Colour, differ also in Degrees of Refrangibility. Newton provided two experiments to support this proposition. These experiments involved the use of prisms, lenses, candles, and red and blue coloured paper. From these experiments, Newton concluded that blue light refracts to a greater degree than red light, and hence that blue light is more refrangible than red light.
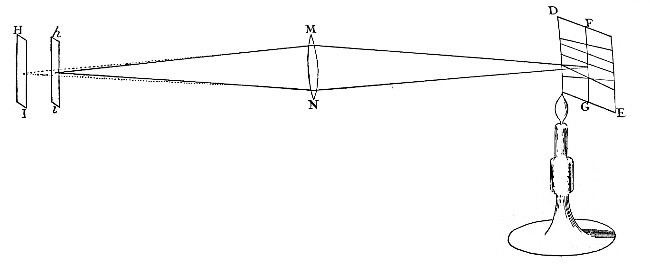
Opticks, part I, figure 12.
In the scholium that followed, Newton pointed out that the red and blue light in these experiments was not strictly homogeneous. Rather, both colours were, to some extent, heterogeneous mixtures of different colours. So it’s not the case, when conducting these experiments, that all the blue light was more refrangible than all the red light. And yet, these experiments demonstrate a general effect. This highlights the fact that, in book 1, Newton was describing ideal experiments in which the target system had been perfectly isolated.
Book 2 concerned the phenomenon now known as ‘Newton’s Rings’: the coloured rings produced by a thin film of air or water compressed between two glasses. It had a different structure to book 1: Newton listed twenty-four observations in part I, then compiled the results in part II, explained them in propositions in part III, and described a new set of observations in part IV. The observations in parts I and IV explored the phenomena of coloured rings in a sequence of increasingly sophisticated experiments.
Consider, for example, the observations in part I. Observation 1 was relatively simple: Newton pressed together two prisms, and noticed that, at the point where the two prisms touched, there was a transparent spot. The next couple of observations were variations on that first one: Newton rotated the prisms and noticed that coloured rings became visible when the incident rays hit the prisms at a particular angle. But Newton steadily progressed, step-by-step, from prisms to convex lenses, and then to bubbles and thin plates of glass. He varied the amount, colour and angle of the incident light, and the angle of observation. The result was a detailed, but open ended, survey of the phenomena.
I have argued that Newton’s experiments and observations cannot be differentiated on the basis of intervention, but there are two other differences worth noting. Firstly, whereas the experiments described in book 1 were ideal experiments, involving perfectly isolated explanatory targets, the observations in books 2 and 3 were not ideal. Rather, through a complex sequence of observations, as the level of sophistication increased, the explanatory target was increasingly well isolated. When viewed in this way, the phenomena of Principia seem to have more in common with the experiments of book 1 than the observations of books 2 and 3.
Secondly, the experiments of book 1 were employed to support particular propositions, and so, individually, they were held to be particularly relevant and informative. In contrast, the observations of books 2 and 3 were only collectively relevant and informative. Moreover, the sequence of observations was open ended: there were always more variations one could try.
What are we to make of these differences between observation and experiment in the Opticks? I have previously argued that, while Newton never constructed Baconian natural histories, his work contained other features of the Baconian experimental philosophy, such as experiments, queries and an anti-hypothetical stance. However, in viewing them as complex, open ended series’ of experiments, I now suggest that the observations of books 2 and 3 look a lot like what Bacon called experientia literata, the method by which natural histories are generated. I’ll discuss this in my next post, but in the mean time, I’d like to hear what our readers think.
Roger Cotes’ ‘Preface’ and the ESD
Peter Anstey writes…
One of the main tasks of this blog over the last three years has been to provide evidence for our claim that from the 1660s the distinction between experimental and speculative philosophy is crucial for an understanding of early modern natural philosophy and even the philosophy of this period in general. More specifically, we have been furnishing evidence that the self-styled experimental philosophers both emphasized the importance of experiment and observation for the acquisition of knowledge, and decried the use of speculation and hypotheses that made little or no appeal to observation. We have also claimed that a prime example of a speculative philosophy that came under attack from experimental philosophers was the Cartesian vortex theory.
It may be surprising, therefore, that hitherto little has been said on this blog about Roger Cotes’ Preface to the second edition of Newton’s Principia published 300 years ago in 1713. For, Cotes’ Preface contains one of the most forthright and sustained defenses of experimental philosophy to be found in the early eighteenth century and it prefaces what can only be described as the most important contribution to natural philosophy in the early modern period.
Cotes begins his Preface with a tripartite distinction between ‘the whole of the Scholastic doctrine derived from Aristotle and the Peripatetics’, (The Principia, 1999, 385) ‘those who take the foundation of their speculations from hypotheses’ and ‘those whose natural philosophy is based upon experiment’. Needless to say, it is this latter method that is ‘incomparably [the] best way of philosophizing’ and ‘which our most celebrated author [Newton] thought should be justly embraced in preference to all others’. (386) The rest of the Preface is a justification of this method of experimental philosophy. First, he elaborates on the method in more detail. He then proceeds to show how Newton’s thesis of universal gravity was established according to this method. Next, he argues against the Cartesian vortex theory and plenist accounts of the universe and, finally, he brings it to a close claiming: ‘Therefore honest and fair judges will approve the best method of natural philosophy, which is based on experiments and observations’. (398).
In this post I shall outline one of the interesting features of Cotes’ critique of the Cartesian vortex theory. In my next post I’ll examine his view of the experimental philosophy in more detail. According to Cotes the speculators ‘are drifting off into dreams, … are merely putting together a romance, elegant perhaps and charming, but nevertheless a romance’ (386) One such romance is the Cartesian vortex theory.
Cometary motion through the vortices of Descartes
In the first edition of the Principia (1687) Newton had advanced a number of arguments against the vortex theory at the end of Book Two, such as the claim that planets moving in a vortex would speed up at the point most distant from the sun when, in fact, the observational evidence and Kepler’s area law showed that they slowed down at this point. But apart from this, little mention is made of the theory. By contrast, in the second edition of the Principia the critique of the vortex theory is a prominent theme. In addition to the arguments at the end of Book Two, the new ‘General Scholium’ appended to the book begins ‘The hypothesis of vortices is beset with many difficulties’ (939) and there follows a whole paragraph on the problems with the theory. The final two sentences deal with the motion of comets, claiming that their regular motion ‘cannot be explained by vortices and that their eccentric motions can only be explained if ‘vortices are eliminated’. These are not claims that Newton makes in the Principia but are rather summaries of arguments that Cotes presents in his Preface.
About one quarter of the Preface is given over to a critique of vortices. In this section, Cotes develops the arguments from cometary motion that are alluded to in Newton’s Scholium. First he claims that bodies in a vortex must move in the same direction and with the same velocity as the surrounding fluid and must have the same density as the fluid that surrounds them. But comets and planets orbit the sun with different velocities and different directions even when they are in the same region of the heavens. Therefore, ‘those parts of the celestial fluid that are at the same distances from the sun revolve in the same time in different directions with different velocities’. But this cannot be accounted for by one vortex, so there will have to be more than one vortex ‘going through the same space surrounding the sun’. It must be asked then ‘how these same vortices keep their integrity without being in the least perturbed through so many centuries by the interactions of their matter’. (394 ) Moreover, because ‘the number of comets is huge’ and they obey the same laws as the planets going ‘everywhere into all parts of the heavens and pass very freely through the regions of the planets, often contrary to the order of the signs … [t]here will be no room at all for the motions of the comets unless that imaginary matter [of the vortices] is completely removed from the heavens’. (395)
What is striking about these arguments is that they are, in effect, the bookends of the Principia. They don’t appear in the body of the work, but are a kind of polemical after thought, and most importantly, they are set within the context of a defense of experimental philosophy. What is it that accounts for the extraordinary fact that Cotes introduced this material in the opening preface and that Newton should allude to it at the end when the arguments are absent from the book? This is not merely a rhetorical question. I would value any comments you may have.
Newton’s ‘Phenomena’ continued…
Kirsten Walsh writes…
In my last post, I considered the phenomena in book 3 of Newton’s Principia. Newton’s decision to label these propositions ‘phenomena’ is puzzling, as they do not seem to fit any standard definition of the term. In this post, I’ll consider Bogen & Woodward’s (1988) distinction between data, phenomena and theories, and suggest that it sheds light both on Newton’s use of ‘phenomena’ and on the connection between his methodology in Opticks and Principia.
Bogen & Woodward (B&W) have argued for a 3-level picture of scientific theories in which:
- ‘Data’ are records produced by measurement and experiment that serve as evidence or features of phenomena. E.g. bubble chamber photographs, and patterns of discharge in electronic particle detectors.
- ‘Phenomena’ are features of the world that in principle could recur under different contexts or conditions. E.g. weak neutral currents, and the decay of a proton.
- ‘Theories’ are explanations of the phenomena.
B&W argue that theories explain phenomena, but not data. Data usually reflect many causal influences besides the explanatory target, while phenomena typically reflect single, or small, manageable numbers of causal influences. For example, General Relativity explains the phenomenon of bending light, but doesn’t explain the workings of the cameras, optical telescopes, etc. that causally influence the data.
Can we characterise Newton’s phenomena in terms of these three levels of theory? Let’s consider phenomenon 1:
- “The circumjovial planets, by radii drawn to the centre of Jupiter, describe areas proportional to the times, and their periodic times – the fixed stars being at rest – are as the 3/2 powers of their distances from that centre.”
In his discussion of this phenomenon Newton explained, “This is established from astronomical observations.” He provided the following table:
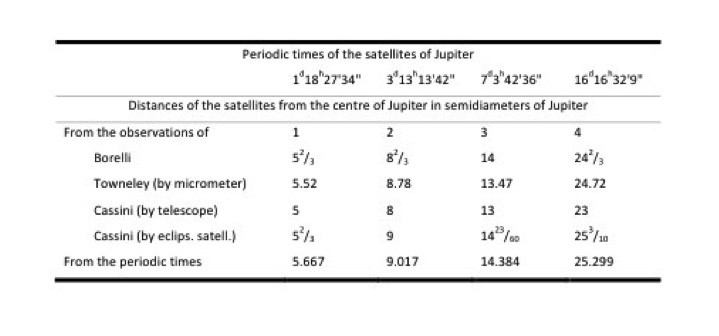These observations are not data in the ‘pure’ sense that B&W discuss. Rather, they are generalisations: average distances and calculated periods of orbit. Moreover, the bottom row contains the average distances calculated from the period and the Harmonic rule (that the periods are as the 3/2 power of the semidiameters of their orbits). These calculations illustrate the ‘fit’ between the expected distance and the observed distance. Nevertheless, they provide a good example of how we might get from a set of data to a phenomenon. So perhaps we can think of them as ‘data’ in a methodological sense: they are records from which phenomenal patterns can be drawn.
I have another reason for considering these calculations ‘data’ in B&W’s sense of the term. In his discussion of phenomenon 1, Newton indicated that these calculations reflect a number of causal influences besides gravity. For instance, he explained that the length of the telescope affected the measurement of Jupiter’s diameter, because
- “the light of Jupiter is somewhat dilated by its nonuniform refrangibility, and this dilation has a smaller ratio to the diameter of Jupiter in longer and more perfect telescopes than in shorter and less perfect ones.”
This is a nice illustration of B&W’s notion of the shift from data to phenomena. By attending to his theory about telescopes, Newton was able to manipulate the data to control for distortion.
Now let’s consider the role of phenomenon 1 in Principia. Phenomenon 1 is employed (in conjunction with proposition 2 or 3, book 1, and corollary 6 to proposition 4, book 1) to support proposition 1, theorem 1, book 3:
- “The forces by which the circumjovial planets are continually drawn away from rectilinear motions and are maintained in their respective orbits are directed to the centre of Jupiter and are inversely as the squares of the distances of their places from that centre.”
This theorem doesn’t contain any information about the sizes or positions of the satellites of Jupiter, or about the workings of telescopes. So, while it explains the phenomenon, it gives no direct explanation of the data. This suggests that, in the Principia, data and phenomena are methodologically distinct.
B&W’s distinction between ‘data’ and ‘phenomena’ reveals two methodological features of Newton’s phenomena:
Firstly, Newton’s phenomena are explananda, but not appearances. Traditionally, ‘phenomenon’ seems to have been synonymous with both ‘appearance’ and ‘explanandum’. For example, the ancient Greeks were concerned to construct a system that explained and preserved the motions of the celestial bodies as they appeared to terrestrial observers. 2000 years later, Galileo and Cardinal Bellarmine argued over which system, heliocentric or geocentric, provided a better fit and explanation of these appearances. This suggests that, traditionally, there was no real difference between phenomena and data. For Newton, however, these come apart. The six phenomena of Principia describe the motions of celestial bodies, but not as they appear to terrestrial observers. In this sense, they are not appearances, but they do require an explanation.
Secondly, this reveals a continuity in Newton’s methodology. The point of Newton’s articulation of ‘phenomena’ in Principia is the same as his experiments in Opticks. Both identify and isolate a pattern or regularity. In the Opticks, Newton isolated his explanatory targets by making observations under controlled, experimental conditions. In Principia, Newton isolated his explanatory targets mathematically: from astronomical data, he calculated the motions of bodies with respect to a central focus. Viewed in this way, Newton’s phenomena and experiments are different ways of achieving the same thing: isolating explananda.
These considerations are admittedly speculative, so I’m keen to hear what our readers think. Does this look like a good way of characterising Newton’s phenomena?
