Birth stats and Divine Providence
Juan Gomez writes…
In a number of posts in this blog we have examined how some philosophers in the eighteenth century were carrying out moral enquiries by following the experimental method that had achieved so much for natural philosophers. The subtitle of Hume’s famous Treatise clearly states the “attempt to introduce the experimental method in morals,” and we know that Turnbull, Butler and Hutcheson were also using this method in their arguments regarding morality, the human mind, and the existence of God. Regarding this latter issue, theistic philosophers like Butler and Turnbull argued that the order and perfection of the natural world (deduced from facts and observation) was clear proof of the wisdom and goodness of God. In this post I want to examine one of such arguments given not by a moral philosopher, but by a famous physician and mathematician: Dr. John Aburthnot.
Dr. Arbuthnot was a fellow of the Royal Society and Physician to the Queen, a fellow Scriblerian of Swift and Pope, a mathematician and a very interesting figure in general. Best known for his work in medicine and his satires, this fascinating polymath wrote a short paper that appeared in the Philosophical Transactions for 1710 titled “An Argument for Divine Providence, Taken from the Constant Regularity Observ’d in the Births of Both Sexes.” He explains how probability works in a situation involving a two-sided dice, and then proceeds to argue that the number of males and females born in England from 1629 to 1710 shows that it was not mere chance, but rather Divine Providence that explains the regularity between the sexes. Let’s examine his argument in more detail.
Arbuthnot begins by considering the purely mathematical aspect of an event where we want to find out the chances of throwing a particular number of two-sided dice (or a coin for that matter). The simplest case is that of 2 coins, where we have that there is one chance of both coins landing on heads, one chance of both coins landing on tails, and two chances where each of the coins lands on a different side. The mathematical details need not detain us here; the main conclusion drawn form this exposition is that the chances of getting an equal number of heads and tails grows slimmer as the number of coins augments. For example, the chances of this happening with ten coins is less than 25%. If instead of coins we consider all human beings which, Arbuthnott assumes, are born either male or female, the chances of there being equal number of each of the sexes are very, very low.
However, Arbuthnot acknowledges that the physical world is not equivalent to the mathematical, and this changes his calculations. If it was just mere chance that operated in the world, the balance between the number of males and females would lean to one or the other, and perhaps even reach extremes. But this is not the case. In fact, or so Arbuthnott argues, nature has even taken into account the fact that males have a higher mortality rate than females, given that the former “must seek their Food with danger…and that this loss exceeds far that of the other Sex, occasioned by Diseases incident to it, as Experience convinces us.” The wisdom of the Author of nature is witnessed in this situation, as the tables of births in England show that every year slightly more males than females are born, in order to compensate for the loss mentioned above and keep the balance. For example in 1629, Arbuthnot’s table list 5218 males to 4683 females; in 1659, 3209 males to 2781 females; in 1709, 7840 males to 7380 females; and so on for all the years recorded.
Arbuthnot concludes that from his argument “it follows, that it is Art, not Chance , that governs,” and adds a scholium where he states that polygamy is contrary to the law of nature.
What can we make of Arbuthnot’s paper? Instead of discussing how effective the argument is (I leave that for the readers to discuss with us in the comments!!), I want to focus on the fact that Arbuthnot’s argument illustrates the call for the use of mathematics in natural philosophy. Philosophers like Arbuthnot and John Keill thought that the use of mathematics had been neglected in natural philosophy and believed that it should play a greater role. From the 1690’s onwards the work of experimental philosphers reveals this use of mathematics in natural philosophical reasoning. The structure of Arbuthnot’s argument resembles that of the natural philosophers who, like Newton, were using mathematics to explain natural phenomena. The mathematical calculation is extrapolated to the case of human births (in this case). Arbuthnot recognizes an issue central to the application of maths in natural philosophy: while the former deals with abstract objects, the latter deals with the natural world. However, in this particular case Arbuthnot uses the asymmetry between the mathematical and physical realms to show that Divine Providence is a better explanation than mere chance when it comes to the balance and regularity of human births. I would like to hear what our readers think of arguments like the one constructed by Arbuthnot.
Cotes’ Preface and Experiment
Peter Anstey writes…
In my last post I introduced Roger Cotes’ famous Preface to the second edition of Newton’s Principia in order to show its importance as an expression of a commitment to experimental philosophy. In that post I focused on Cotes’ critique of the Cartesian vortex theory and the manner in which this attack on the archetypal speculative philosophy formed the bookends of the Principia. In this post I will discuss the role of experiment in Cotes’ comments on experimental philosophy.
The Preface is actually quite a complex essay that has both polemical and expository agendas. On the one hand, Cotes uses it to give a summary of the main theses of the Principia centred around Newton’s theory of gravity. On the other hand, Cotes uses it to defend the theory of gravity against the charge that it is an occult quality, to defend Newton’s system of the world against the Cartesian vortex theory, and to defend the methodology of the work against rival approaches.
On this latter point, Cotes begins by claiming that Newton’s method is ‘based upon experiment’ (The Principia, eds I.B. Cohen and A. Whitman, Berkeley: University of California Press, 1999, p. 386). One might expect here that Cotes will give a list of the sorts of experimental results that Newton achieved or some reference to crucial experiments, but instead he introduces another set of methodological notions: phenomena, principles, hypotheses, analysis and synthesis. It is only later when appealing to various laws, principles and axioms in his summary of Newton’s system of the world that Cotes refers to experiments.
Here is a summary of Cotes’ account of the method of the Principia. Natural philosophy attempts to derive the causes of all things from the simplest of principles and not from contrived hypotheses. These principles are derived from the phenomena by a two-step process of analysis and synthesis. From select phenomena the forces and simpler laws of these forces are ‘deduced’ by analysis. Then by synthesis ‘the constitution of the rest of the phenomena’ is given. In the case of the Principia the relevant force is gravitational attraction and the relevant law is the inverse square law. Though Cotes throws in the laws of planetary motion claiming that ‘it is reasonable to accept something that can be found by mathematics and proved with the greatest certainty’ (p. 389). He also claims, after presenting a summary of the system of the world, ‘the preceding conclusions are based upon an axiom which is accepted by every philosopher, namely, that effects of the same kind –– that is, effects whose known properties are the same –– have the same causes, and their properties which are not yet known are also the same’. Indeed, ‘all philosophy is based on this rule’ (p. 391).
Where then do experiments fit in this picture? The first mention of experiments is in relation to the law of fall. Cotes refers here to pendulum experiments and to Boyle’s air-pump. Next, Huygens’ pendulum experiments are referred to in the discussion of the determination of the centripetal force of the moon towards the centre of the Earth (p. 389). They then appear in the elaboration of the ‘same effect, same cause’ axiom and its application to the attribution of gravity to all matter. Cotes says ‘[t]he constitution of individual things can be found by observations and experiments’ and from these we make universal judgments (p. 391). Thus, ‘since all terrestrial and celestial bodies on which we can make experiments or observations are heavy, it must be acknowledged without exception that gravity belongs to all bodies universally. … extension, mobility, and impenetrability of bodies are known only through experiments’ and so too is gravity. Finally, in recapping the Newtonian method near the conclusion of the Preface Cotes repeats that ‘honest and fair judges will approve the best method of natural philosophy, which is based on experiments and observations’ (p. 398).
What are we to make of the role of experiments here? First, notice how experiments are appealed to in the establishment of laws and the ‘same effect, same cause’ axiom. Second, it is worth pointing out that the ‘same effect, same cause’ axiom is Newton’s second rule of philosophizing: indeed, Cotes uses the very same example as Newton, namely, the falling of stones in America and Europe (see p. 795). Third, notice how without any explanation Cotes extends experiments to experiments and observations. He begins by saying that there are those ‘whose natural philosophy is based on experiment’ and he ends by saying that ‘the best method of natural philosophy, … is based on experiments and observations’. This is not an equivalent expression and while it is consistent with many other methodological statements by experimental philosophers, it still calls out for explanation.
Has Cotes really given an adequate summary of the method of experimental philosophy and has he captured the manner in which experiments are used in Newton’s reasoning in the Principia? In my view he has not. I’d be interested to hear your views?
Cartesianism, experimentalism, and the experimental-speculative distinction
A guest post by Tammy Nyden and Mihnea Dobre.
Tammy Nyden and Mihnea Dobre write…
A while ago, we published an announcement on this blog of our forthcoming edited volume, Cartesian Empiricisms (Springer 2013). A claim in that post – that some Cartesians “seem to escape the ESD distinction” – has been questioned by Peter Anstey in another post. We thank him for the intervention and would like to push forward our claim and discuss it in more detail as this will reveal some of our concerns with the ESD (experimental-speculative distinction).
In his reply, Peter Anstey asked, “Did the Cartesians practise a form of experimental philosophy analogous to that of the Fellows of the early Royal Society?” We would argue that the question itself is problematic, as there are not two practices or worldviews to compare. There is variation among the Cartesians as well as among the fellows of early Royal Society. In order to gain a nuanced understanding of these historical actors, we suggest a rather different question: “What role did Cartesian philosophy play in the acceptance and spread of experimental practices in late seventeenth-century philosophy?” When we ask this question, we recognize the experiments of Robert Desgabets on blood transfusion, Henricus Regius on liquids, Burchard de Volder’s with air-pumps, etc., and consider how their work improved experimental technologies, influenced a theoretical reflection on the role of experiments and the senses in natural philosophy, and influenced institutional change that was favorable to experimental science.
Because Cartesians took various aspects of Descartes’ system and merged it with various aspects of experimentalism, there is not one ‘Cartesian’ use of experiment, but several. For example, both Regius and de Volder promoted experiment, but Regius rejects Descartes’ theory of innate ideas while de Volder defends it. Many Cartesians came to reject hyperbolic doubt, some defended vortex theory, some did not. Cartesian Empiricisms is not a complete inventory of such views expressed by Descartes’ followers. Rather our goal was to encourage the discussion of the above-mentioned question and to reveal some aspects that have been unfortunately neglected so far by both historians of philosophy and science.
Readers of this blog are familiar with the objection that traditional historiography of science was built on the Rationalist-Empiricist distinction (RED). A consequence is the exclusion of so-called “rationalists” from the histories of science, particularly history of the use, development and acceptance of experiment. This is problematic because recent research (e.g., Ariew, Lennon and Easton, Easton, Schmaltz, Cook, Nyden, Dobre, etc.) shows that many so-called rationalists were deeply involved in the practice and spread of the acceptance of experiment in natural philosophy. Cartesian Empiricisms gives further emphasis to this issue, as it examines several philosophers who identified as committed Cartesians who were deeply involved in experiment. According to historiographies that divide the period into two mutually exclusive epistemologies or methodologies these philosophers either do not exist (i.e., they are overlooked by histories of philosophy and science) or are seen as “not really Cartesian” or “not really experimentalist,” as it would be needed by that particular narrative. Thus, we do share the concern of the authors of this blog, that such binaries as RED force us to fit philosophers into categories that they would not themselves recognize and causes us to misrepresent seventeenth-century natural philosophy. Moreover, we acknowledge that this blog importantly shows the anachronism of the RED, a way of viewing the period that is constructed later by what may be called Kantian propaganda. However, we would like to raise now some of our concerns with the distinction promoted by this blog, the experimental-speculative distinction (ESD) and explain why some Cartesians would escape the ESD. Our worries cover two important aspects of the ESD: the label “speculative” and the actor-category problem.
(1) In a very recent post, Peter Anstey argued that eighteenth-century Newtonians pointed out Cartesian vortex theory as a prime representative of speculative philosophy (our emphasis). We caution against letting eighteenth-century Newtonian propaganda color a historical interpretation of seventeenth-century natural philosophy. Voltaire, d’Alembert and others took great pains to contrast Newtonianism from Cartesianism as two mutually exclusive worldviews who battled it out, with Newton’s natural philosophy as the victor. But the reality is that after Descartes’ death (1650) and before the victory of Newtonianism in the middle of the eighteenth century, followers of both Descartes and Newton had more in common than we are led to believe. More importantly, both “camps” had more diversity than we were ready to accept in the traditional histories. Cartesian Empiricisms draws attention to that diversity within Cartesianism. Perhaps the one thing Cartesians discussed in the chapters of this volume do have in common is that they do both experimental and speculative philosophy, as these two categories are sometimes defined on this blog. But this last claim leads to our second concern with the ESD.
(2) A reader of this blog will find that when ESD is compared to RED, the first advantage highlighted over the latter is that “the ESD distinction provided the actual historical terms of reference that many philosophers and natural philosophers used from the 1660s until late into the 18th century.” While there is no doubt that many early modern philosophers were using this language (i.e., “experimental” and “speculative”) in their writings, it is equally true that such language is not in use by the Cartesians. If one would be very strict with picking up “the actual historical terms of reference,” one will see another pair of terms keep mentioned by various Cartesians, “experience” and “reason.” Of course, one can read this pair as another form of the ESD, but that would be an interpretation, and a problematic one at that. Both the Cartesians and the so-called “experimentalists” were trying to determine the proper relationship between reason and experience and when one looks at their attempts, it becomes even more difficult to draw a clear line between speculative philosophers and experimentalist philosophers.
Our concern is the possible danger of transforming ESD into a new RED. Experimental and speculative may be useful adjectives to describe aspects of a particular philosophy or particular commitments of a philosopher (especially when the two terms are clearly stated in one’s writings). However, they are not useful for dividing philosophers or their natural philosophies, particularly when they are not already conceived as falling within the “experimental philosophy” camp, as is the case for Cartesians at the end of the seventeenth century.
Newton’s ‘Phenomena’ continued…
Kirsten Walsh writes…
In my last post, I considered the phenomena in book 3 of Newton’s Principia. Newton’s decision to label these propositions ‘phenomena’ is puzzling, as they do not seem to fit any standard definition of the term. In this post, I’ll consider Bogen & Woodward’s (1988) distinction between data, phenomena and theories, and suggest that it sheds light both on Newton’s use of ‘phenomena’ and on the connection between his methodology in Opticks and Principia.
Bogen & Woodward (B&W) have argued for a 3-level picture of scientific theories in which:
- ‘Data’ are records produced by measurement and experiment that serve as evidence or features of phenomena. E.g. bubble chamber photographs, and patterns of discharge in electronic particle detectors.
- ‘Phenomena’ are features of the world that in principle could recur under different contexts or conditions. E.g. weak neutral currents, and the decay of a proton.
- ‘Theories’ are explanations of the phenomena.
B&W argue that theories explain phenomena, but not data. Data usually reflect many causal influences besides the explanatory target, while phenomena typically reflect single, or small, manageable numbers of causal influences. For example, General Relativity explains the phenomenon of bending light, but doesn’t explain the workings of the cameras, optical telescopes, etc. that causally influence the data.
Can we characterise Newton’s phenomena in terms of these three levels of theory? Let’s consider phenomenon 1:
- “The circumjovial planets, by radii drawn to the centre of Jupiter, describe areas proportional to the times, and their periodic times – the fixed stars being at rest – are as the 3/2 powers of their distances from that centre.”
In his discussion of this phenomenon Newton explained, “This is established from astronomical observations.” He provided the following table:
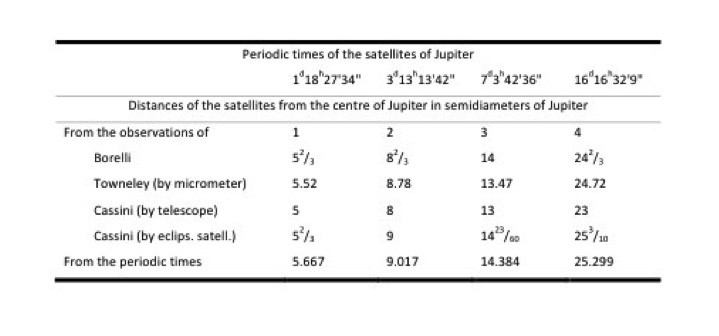These observations are not data in the ‘pure’ sense that B&W discuss. Rather, they are generalisations: average distances and calculated periods of orbit. Moreover, the bottom row contains the average distances calculated from the period and the Harmonic rule (that the periods are as the 3/2 power of the semidiameters of their orbits). These calculations illustrate the ‘fit’ between the expected distance and the observed distance. Nevertheless, they provide a good example of how we might get from a set of data to a phenomenon. So perhaps we can think of them as ‘data’ in a methodological sense: they are records from which phenomenal patterns can be drawn.
I have another reason for considering these calculations ‘data’ in B&W’s sense of the term. In his discussion of phenomenon 1, Newton indicated that these calculations reflect a number of causal influences besides gravity. For instance, he explained that the length of the telescope affected the measurement of Jupiter’s diameter, because
- “the light of Jupiter is somewhat dilated by its nonuniform refrangibility, and this dilation has a smaller ratio to the diameter of Jupiter in longer and more perfect telescopes than in shorter and less perfect ones.”
This is a nice illustration of B&W’s notion of the shift from data to phenomena. By attending to his theory about telescopes, Newton was able to manipulate the data to control for distortion.
Now let’s consider the role of phenomenon 1 in Principia. Phenomenon 1 is employed (in conjunction with proposition 2 or 3, book 1, and corollary 6 to proposition 4, book 1) to support proposition 1, theorem 1, book 3:
- “The forces by which the circumjovial planets are continually drawn away from rectilinear motions and are maintained in their respective orbits are directed to the centre of Jupiter and are inversely as the squares of the distances of their places from that centre.”
This theorem doesn’t contain any information about the sizes or positions of the satellites of Jupiter, or about the workings of telescopes. So, while it explains the phenomenon, it gives no direct explanation of the data. This suggests that, in the Principia, data and phenomena are methodologically distinct.
B&W’s distinction between ‘data’ and ‘phenomena’ reveals two methodological features of Newton’s phenomena:
Firstly, Newton’s phenomena are explananda, but not appearances. Traditionally, ‘phenomenon’ seems to have been synonymous with both ‘appearance’ and ‘explanandum’. For example, the ancient Greeks were concerned to construct a system that explained and preserved the motions of the celestial bodies as they appeared to terrestrial observers. 2000 years later, Galileo and Cardinal Bellarmine argued over which system, heliocentric or geocentric, provided a better fit and explanation of these appearances. This suggests that, traditionally, there was no real difference between phenomena and data. For Newton, however, these come apart. The six phenomena of Principia describe the motions of celestial bodies, but not as they appear to terrestrial observers. In this sense, they are not appearances, but they do require an explanation.
Secondly, this reveals a continuity in Newton’s methodology. The point of Newton’s articulation of ‘phenomena’ in Principia is the same as his experiments in Opticks. Both identify and isolate a pattern or regularity. In the Opticks, Newton isolated his explanatory targets by making observations under controlled, experimental conditions. In Principia, Newton isolated his explanatory targets mathematically: from astronomical data, he calculated the motions of bodies with respect to a central focus. Viewed in this way, Newton’s phenomena and experiments are different ways of achieving the same thing: isolating explananda.
These considerations are admittedly speculative, so I’m keen to hear what our readers think. Does this look like a good way of characterising Newton’s phenomena?
René Réaumur and Charles Dufay on Experimental Natural Philosophy
A guest post by Michael Bycroft, a PhD Student at Cambridge.
Michael Bycroft writes…
René Réaumur
In a recent post Peter Anstey asked: “When did the French embrace experimental philosophy?” In this post I want to do two things. One is to draw attention to two Frenchmen who practised experimental natural philosophy (ENP) well before Jean-Antoine Nollet began teaching this method in the mid-late 1730s. These men were René Réaumur and Charles Dufay. My other task is to try to explain why these men, who did so much to practice ENP, did so little to explicitly define or defend their practice.
René Réaumur (1683-1757) was arguably the most active and influential member of the Académie des Sciences in the first half of the eighteenth century. Nowadays he is known for his research on insects, steel-making, and thermometry, but his interests were truly encyclopaedic. Charles Dufay (1698-1739) is known to historians of physics as a student of electricity, but his research interests were nearly as broad as those of Réaumur, his patron and collaborator.
There is no doubt that these two men practiced ENP. It is true that they were Cartesians, in the sense that their chief theoretical resources were vortices and subtle fluids. But they wore their theory lightly, and they saw themselves primarily as experimenters rather than as system-builders. This pair was at least as committed to ENP, and in some cases more so, than their French colleague Nollet or their English counterparts Francis Hauksbee the Elder and John Desaguliers.
Yet it is hard to find clear, succinct, accessible endorsements of the key tenets of ENP in the writings of Dufay and Réaumur. Such endorsements do exist, but they are invariably buried in the middle of one or other of the many papers they published in the Académie’s journal, the Mémoires de l’Académie Royale des Sciences. Here is an example from one of Réaumur’s first papers, on the growth of shells, published in the 1709 volume of the Mémoires:
- But conjectures such as these [ie. the ones Réaumur had just advanced in the first part his paper] are not enough in true natural philosophy. Experiments performed on the matters at hand are the only sound basis for our reasoning…It is to experiments that I shall turn to decide whether I have correctly described the manner in which nature behaves, or whether [instead] everything I have said is merely a trick of the imagination.
- Mais de pareilles conjectures ne suffisent point en bonne Physique. Les seules expériences faites sur les choses dont il est question, y doivent servir de bases à nos raisonnemens. … C’est aux experiences que je vais rapporter à faire voir, si j’ai véritablement décrit la maniere dont la Nature agit, ou si l’on doit regarder tout ce qu’on vient d’avancer comme un simple jeu d’imagination.
Charles Dufay
This statement is clearly in the spirit of ENP, and similar statements can be found elsewhere in Réaumur’s papers, and in Dufay’s. But they are fleeting asides rather than stand-alone manifestos. Why were these men so reticent?
An important part of the answer is that the stand-alone manifestos of Nollet, Hauksbee and Desaguliers appear in the prefaces of their natural philosophy textbooks, and Dufay and Réaumur did not write textbooks. They did not need to. They were independently wealthy, drew sizeable pensions from the Academy, and were well-rewarded by the state for their research on French industries such as steel and textiles.
Perhaps it is also relevant that Bernard le Bovier de Fontenelle, the Perpetual Secretary of the Academy, did much to define and defend the Academy’s activities on behalf of its members.
Another factor may be that Dufay and Réaumur were more concerned to defend the application of natural philosophy to industry (against skeptical artisans and ministers) than they were to defend the application of experiment to natural philosophy (against speculative philosophers). At any rate, the former concern dominated the preface to Réaumur’s first book, L’art de convertir le fer en acier (1722).
Finally, as we have seen, Dufay and Réaumur dispensed methodological advice in the course of the papers they published in the Academy. Perhaps they considered this the best forum for expressing their views on ENP, even though this choice makes their views harder for the historian to identify than if they had written textbooks or dictionary entries instead.
This is not to say that Dufay and Réaumur had no connections with earlier and later textbook writers on ENP in France. On the contrary. They both learned much of their physics from Jacques Rohault’s Traité de physique, and in their turn they taught Nollet much of what he knew about experimentation (Nollet assisted both Dufay and Réaumur in their laboratories in the early 1730s). These connections reinforce the broader lesson of this post, which is that the leading practitioners of ENP were not always its most explicit promoters.
Defining Early Modern Experimental Philosophy (2)
Alberto Vanzo writes…
In my last post, I raised the question as to whether there is any methodological view that was shared by all or most early modern experimental philosophers. To paraphrase Bas Van Fraassen, is there any statement E+ such that
- To endorse the method of (early modern) experimental philosophy = to believe that E+ (the experimentalists’ methodical dogma)?
As those of you who have followed this blog for a while will know, early modern experimental natural philosophers claimed that we should reject hypotheses and speculations (that is, roughly, natural-philosophical claims and theories) and rely instead on experiments and observations. In this post, I will discuss whether this claim, suitably understood, is the experimentalists’ methodical dogma. What does their rejection of hypotheses amount to?
The statement that we should reject hypotheses does not mean that we should avoid learning natural-philosophical claims and theories. On the contrary, according to Robert Hooke, learning hypotheses is beneficial because it helps us to devise new explanations and raise questions:
- the Mind will be somewhat more ready at guessing at the Solution of many Phenomena almost at first Sight, and thereby be much more prompt at making Queries, and at tracing the Subtilty of Nature, and in discovering and searching into the true Reason of things […]
Experimental philosophers also allow us to entertain claims and theories for the sake of testing them. Robert Boyle states in a letter to Oldenburg that natural histories should include “Circumstances” such that their “tryal or Observation” is “necessary or sufficient to prove or to invalidate this or that particular Hypothesis or Conjecture”.
Boyle’s statement makes clear that he allows for the acceptance of a natural-philosophical claims that are proven by “tryal [experiment] or Observation”. The claims in question must be those that are expressed by substantive or – in Kantian terms – synthetic a posteriori statements. Experiments and observations cannot prove analytic a priori statements. These are hardly the kind of statements that concerned experimental philosophers. Assuming that the analytic/synthetic distinction is tenable, accepting analytic a priori statements as true seems to be a harmless move anyway.
In the light of this, we may be tempted to paraphrase the rejection of hypotheses as follows:
- [A] Only commit to those substantive (as opposed to analytic) claims and theories that are warranted by experiments or observations.
[A] is in line with experimental philosophers’ rejection of arguments from authority, epitomized by the motto of the Royal Society: “nullius in verba“, which can be loosely translated as “take no man’s word for it”. [A] entails the rejection not only of arguments from authority, but also any kind of a priori arguments for substantive natural-philosophical claims – for instance, the arguments that Descartes used in the Principles of Philosophy to establish that material objects are made up of corpuscles. [A] has the welcome effect of classifying Descartes where, in my view, he belongs: outside of the movement of experimental philosophy, even though he too gathered natural-philosophical observations and performed some experiments.
However, [A] is inconsistent with the fact that many experimental philosophers were committed to substantive claims, like the corpuscularian and mechanist hypotheses, that were hardly warranted by the then extant empirical evidence. Boyle or Montanari did not seem to be concerned to provide detailed empirical arguments for corpuscularism or mechanism. However, they did not regard their acceptance of these views as being inconsistent with their commitment to experimentalism.
In view of this, I suggest replacing [A] with [B]:
- [B] Only firmly commit to those substantive claims and theories that are warranted by experiments and observations
and claiming that experimental philosophers like Boyle and Montanari did not firmly commit to corpuscularism and mechanism. They only weakly, tentatively, provisionally commit to these views, even though they were confident that future discoveries would dispel any doubt on their truth.
Is it correct to say that experimental philosophers’ commitments to mechanism and corpuscularism was typically weak, provisional, tentative? Are there other claims on the natural world that experimental philosophers firmly endorsed, even though the then available empirical evidence did not warrant them? Can a clear distinction between weak, provisional, tentative and strong, definitive, firm commitments be drawn, and if so, how? If you have any suggestions on how these questions should be answered, please let me know in the comments or get in touch. Answering these questions is important to establish if my suggestion that [B] represents a suitable candidate for the experimentalists’ methodical dogma is persuasive.
Teaching Experimental Philosophy IV: the case of John Keill
Peter Anstey writes …
In my last post we met the instrument maker and promoter of experimental philosophy Francis Hauksbee the Elder. Hauksbee, however, wasn’t the first lecturer to give public lectures in England on the exciting new developments in natural philosophy. That honour rests with a Scotsman called John Keill.
John Keill (1671–1721) came under the tutelage of the first Newtonian David Gregory in Edinburgh. He followed Gregory to Oxford in 1691 and by 1699 was giving lectures. Around 1704/95, according to his student John Theophilus Desaguliers, Keill became ‘the fi
rst who publickly taught Natural Philosophy by Experiments in a mathematical Manner’ (A Course of Experimental Philosophy, Volume 1, 1734, Preface). His lectures were published in Latin in 1702 and in English translation in 1720 under the title of An Introduction to Natural Philosophy: or, Philosophical Lectures read in the University of Oxford Anno Dom. 1700.
It is not clear, however, that Keill saw himself as teaching experimental philosophy. Some scholars have claimed that Keill was appointed as a lecturer in experimental philosophy at Oxford in 1704 and that he was the first to teach experimental philosophy there. Indeed, in 1707 The Oxford Intelligencer advertised his ‘Course of Mechanical and Experimental Philosophy’. Moreover, in the preface to his Introduction to Natural Philosophy he does express his opposition to speculative natural philosophy, particularly Cartesianism, singling out the Cartesian theory of gravity for particularly harsh treatment (pp. iv–vii).
Hence, one might naturally assume that he is a straightforward advocate of experimental philosophy, and yet this is not the case. For, in the first lecture Keill proceeds to distinguish four ‘Sects of Philosophers’: the Pythagoreans and Platonists; the Peripatetics; those who ‘proceed upon Experiments; and the Mechanical’ (pp. 1–3). He then informs the reader that ‘Amongst these various ways of Philosophizing, there is no particular one, wherein we do intirely acquiesce’ (p. 3). In fact, Keill saw himself as pursuing, not the new experimental philosophy, but what he calls ‘Mathematical Philosophy’ inspired by Newton and characterized by ‘applying Geometry to Natural Philosophy’.
As for experimental philosophy, Keill warns that:
- many of the Experiments that the third Sect of Philosophers [experimental philosophers] have delivered down to us, must be made use of: tho this ought not to be done without great Caution; for we are well apprised how fond these Gentlemen are of their Theories, how willing they are that they should be true, and how easily they deceive both others and themselves, in trying their Experiments (p. 7).
It is clear from this passage that Keill’s conception of what constitutes an experimental philosopher differs from that of Boyle and others, for Keill finds them too fond of their theories, whereas what characterises the experimental philosophers throughout the latter decades of the seventeenth century is their extreme caution in making any theoretical commitments until the observational and experimental data is assembled. Keill’s experimental philosopher would be foreign to most who aligned themselves with the movement.
The method that Keill follows instead is that of ‘The great Philosopher of this age, the most Ingenious and Incomparable Mr. Newton’ who ‘by his great and deep skill in Geometry’ was able to show the inconsistencies of Descartes’ vortex theory. Keill’s opponents in natural philosophy were not the speculative philosophers but ‘our ungeometrical Philosophers’ (p. 24). Thus Keill is representative of the first generation of those, like John Arbuthnot and John Harris who, inspired by Newton, adopted a straightforwardly mathematical approach to natural philosophy. Surprisingly, Keill’s reservations about experimental philosophy were completely ignored by the likes of Hauksbee the Elder and Desaguliers who preferred to see their efforts in promoting experimental philosophy as following Keill’s example and, in Desaguliers’ case, even recycling some of his lectures.
Newton on Experiment and Mathematics
Kirsten Walsh writes…
In my last post, I discussed our 20 revised theses and why I altered thesis 5. In this post, I’ll discuss why I replaced thesis 8.
In 2011, I claimed that:
- 8. The development of Newton’s method from 1672 to 1687 appears to display a shift in emphasis from experiment to mathematics.
But at the start of this year, I replaced this thesis with a new thesis 8:
- 8. In his early work, Newton’s use of the terms ‘hypothesis’ and ‘query’ are Baconian. However, as Newton’s distinctive methodology develops, these terms take on different meanings.
Since my new thesis is a replacement of the original thesis, rather than a modification, two explanations are required. So in today’s post, I’ll tell you why I decided to remove my original thesis 8, and in my next post, I’ll tell you about my new thesis 8.
I originally included thesis 8 because there are some obvious differences in the styles of Newton’s early work on optics and his Principia. In Newton’s first paper on optics (1672), there is a strong emphasis on experiment. Experiment drives his research and guides his rejection of various possible explanations of the phenomena under consideration. Ultimately, he presents an Experimentum Crucis as proof for the certainty of his proposition that white light is heterogeneous. In contrast, the Principia (1687) displays a strong emphasis on mathematics. The full title of the work, the Author’s Preface to the Reader, and the fact that Book I opens with 11 lemmas outlining the mathematical framework of the work are just a few features that make it clear that Principia is primarily a mathematical treatise.
I now think that my original thesis 8 is misleading.
Firstly, as I have emphasised on this blog, Newton’s early work had a mathematical style that made it unique among his contemporaries. While they recognised him as an experimental philosopher, his claims of obtaining certainty via geometrical proofs set him apart from the Baconian-experimental philosophers. Moreover, his methodological statements show evidence of a tension between experiment and mathematical certainty. For example, he says that the science of colours,
- “depend[s] as well on Physicall Principles as on Mathematicall Demonstrations: And the absolute certainty of a Science cannot exceed the certainty of its Principles. Now the evidence by wch I asserted the Propositions of colours is in the next words expressed to be from Experiments & so but Physicall: Whence the Propositions themselves can be esteemed no more then Physicall Principles of a Science.”
Secondly, Newton continued to identify as an experimental philosopher until the end of his life. For example, in the General Scholium at the end of Principia, he says:
- “and hypotheses, whether metaphysical or physical, or based on occult qualities, or mechanical, have no place in experimental philosophy.”
This resembles Newton’s earlier emphasis on grounding propositions on empirical evidence, rather than on speculative conjectures.
Thirdly, in Principia, Newton appears to be negotiating a similar tension between experiment and mathematical certainty that we saw in his early work. For example, in the Scholium to the Laws of Motion he asserts the certainty of his Laws, while at the same time, acknowledging their experimental basis:
- “The principles I have set forth are accepted by mathematicians and confirmed by experiments of many kinds.”
And:
- “By these examples [i.e. the experiments mentioned above] I wished only to show the wide range and the certainty of the third law of motion.”
From these three points, we can see that the methodological differences between Newton’s early papers and Principia aren’t as great as they first appear. But I did not remove my original thesis 8 because I think that the methodology of the 1672 paper is precisely the same as the methodology displayed in Principia. Rather, I don’t think my original thesis 8 captures what is important about these differences.
As I have explained here, my project is to distinguish between those features of Newton’s methodology that changed, and those that stayed the same. Some aspects of Newton’s methodology developed over time. For example, he came to value geometrical synthesis over algebraic analysis. Other aspects of his methodology varied according to context. For example, in Opticks, he employs ‘experiments’ and ‘observations’, but in Principia, he employs ‘phenomena’. But this triumvirate of methodological ideas – experiment, mathematics and certainty – should be considered an enduring feature of Newton’s methodology.
Joseph Butler and Method in Moral Philosophy and Religion
Juan Gomez writes…
One of the features that we have explored in our project is the application of the experimental method in areas other than natural philosophy. I have focused on the work of George Turnbull to illustrate how the method was applied in moral philosophy and religion. In my next series of posts I want to focus on the work of Joseph Butler (who was arguably one of the figures that most influenced Turnbull) and explore his ideas on method in moral and religious inquiries.
As an introductory post to this series I will focus on the preface that appeared in the 1788 edition of Butler’s Analogy of Religion (first edition 1736) by Samuel Halifax, Bishop of Gloucester. In this preface Halifax gives an account of Butler’s ‘moral and religious systems’ that serves as a summary of Butler’s main ideas.
Halifax tells us that Butler’s moral system can be found mainly in the first three of his collection of Sermons, which are on human nature. Halifax highlights the way Butler proceeds to determine human nature:
What the inward frame and constitution of man is, is a question of fact; to be determined, as other facts are, from experience, from our internal feelings and external senses, and from the testimony of others…From contemplating the bodily senses, and the organs or instruments adapted to them, we learn that the eye was given to see with, the ear to hear with. In like manner, from considering our inward perceptions and the final causes of them, we collect that the feeling of shame, for instance, was given to prevent the doing of things shameful; compassion, to carry us to relieve others in distress; anger, to resist sudden violence offered to ourselves.
The method for acquiring knowledge of our moral system must be the same we employ to discover external facts. Halifax contrasts this method that Butler adhered himself to with the one used by Samuel Clarke. Halifax and Butler both see the two methods not as opposed to each other but rather as complementary:
The reader will observe, that this way of treating the subject of morals, by an appeal to facts, does not at all interfere with that other way, adopted by Dr. Samuel Clarke and others, which begins inquiring into the relations and fitness of things, but rather illustrates and confirms it.
It is interesting that Clarke is portrayed as following an a priori method in his moral inquiries, and I will leave the analysis of this and its relation to Butler’s method for a future post where we will examine the correspondence between Butler and Clarke regarding this issue. Since this is just an introductory post, I want to finish by showing Halifax’s rendering of Butler’s method in his religious work.
Butler’s main philosophical text was his Analogy, where he deals with natural and revealed religion and argues that the former is confirmed in the latter, both giving us evidence for the divine government of the world. The way Butler proceeds in this text is by arguing by analogy from the natural to the moral realm:
This way of arguing from what is acknowledged to what is disputed, from things known to other things that resemble them, from that part of the divine establishment which is exposed to our view to that more important one which lies beyond it, is all on hands confessed to be just. By this method Sir Isaac Newton has unfolded the system of nature; by the same method Bishop Butler has explained the system of grace; and thus, to use the words of a writer, whom I quote with pleasure, has ‘formed and concluded a happy alliance between faith and philosophy.’
One of the advantages of this method of analogy is that (as we will see in my next post where we examine Butler’s Analogy in a bit more detail) it does not lead to a claim of certain knowledge about the doctrines of religion, but it rather leads to a high degree of credibility in them. This feature allows Butler to get rid of a number of objections. Further, if the analogy Butler argues for is a strong one, then all objections made against revealed religion will also count as objections to natural religion, which is a consequence the objectors will not want to admit.
As we have seen in this brief account of Halifax’s preface, it appears that a striking feature of Butler’s work in morality and religion is the methodology he applies in both areas, one that argues from facts and experience instead of hypotheses and speculations:
Instead of indulging to idle speculations, how the world might possibly have been better than it is; or, forgetful of the difference between hypothesis and fact, attempting to explain the divine economy with respect to intelligent creatures, from preconceived notions of his own; he [Butler] first inquires what the constitution of nature, as made known to us in the way of experiment, actually is; and from this, now seen and acknowledged, he endeavours to form a judgment of that larger constitution, which religion discovers to us.
In my next post we will examine Butler’s application of this method in his Analogy and his defense of probable knowledge.
Newton and the ESD
Kirsten Walsh writes…
We rang in 2013 by reconsidering our set of 20 core theses on the emergence and fate of early modern experimental philosophy. While our general theses regarding the distinction between experimental and speculative philosophy (ESD) were unchanged, I altered several of the specific claims about Newton’s methodology. In this post, I’ll focus on thesis 5 and why I changed it.
In 2011, I claimed that:
5. The ESD is operative in Newton’s early optical papers.
By ‘operative’, I mean that Newton appears to frame his methodology in terms of the ESD and aligns himself with the experimental philosophers of the Royal Society. While Newton’s methodology differed from his contemporaries in important ways (for example, unlike his contemporaries, Newton emphasised quasi-mathematical reasoning), it nevertheless reflects some of the key ideas and preferences of the Royal Society. Previously, I have discussed Newton’s early anti-hypothetical stance and Newton’s early use of queries as evidence of this his preference for the experimental philosophy of the Royal Society.
I now have enough evidence to broaden the scope of thesis 5. The ESD is operative in all of Newton’s scientific work; not just his early work:
5. The ESD is operative in Newton’s work, from his early work on optics in the 1670s to the final editions of Opticks and Principia published in the 1720s.
Let’s start with Newton’s Opticks. This book is widely recognised as a work of experimental philosophy. Newton’s experimental focus is made explicit by the opening sentence (which appears in every edition):
- “My Design in this Book is not to explain the Properties of Light by Hypotheses, but to propose and prove them by Reason and Experiments…”
Moreover, the presence of queries and the absence of hypotheses reflect the epistemic commitments of the experimental philosophy.
Commentators often notice that, in later editions of Opticks, Newton’s queries become increasingly speculative. This suggests that, despite his use of ESD-jargon, Newton was not following the experimental philosophy after all. In response to this kind of objection, I have argued that these later queries perform a role that is distinct to that of hypotheses, and that this role is consistent with Newton’s methodology. Moreover, the general features of Newton’s methodology reflect his commitment to experimental philosophy in opposition to speculative philosophy. In short, the ESD is operative in every edition of the Opticks.
Now consider Newton’s Principia. This book is often seen as less a work of experimental philosophy and more a work of mathematics. However, I have argued that the methodological passages in the first edition of Principia, though sparse, make it clear that experiment is an important theme of this work. Moreover, in the ‘General Scholium’, which was introduced in the 2nd edition in 1713, Newton makes his commitment to the experimental philosophy explicit.
Commentators often notice that Newton’s use of hypotheses in Principia, and their changing roles between the three editions, suggest that his methodology changes over time. However, I have argued that, in all three editions, Newton’s use of hypotheses is consistent with his experimental method. Moreover, the late introduction of Rule 4 in 1726 demonstrates that this commitment to experimental philosophy, in opposition to speculative philosophy, is long-lasting. In short, the ESD is operative in every edition of the Principia.
To summarise, the notions of experiment, queries and a decrying of speculative hypotheses that are enduring themes in Newton’s work, from the 1670s to his death in 1727, support my broader thesis 5. Commentators often see Newton’s use of these notions as rhetorical and argue that he failed to follow his own methodology. However, I argue that Newton’s methodology is internally consistent. Moreover, these methodological statements are more than ‘mere’ rhetoric. Rather, to some extent they track his epistemic and ontological commitments.
Do you think my argument is convincing? I’d love to hear what you think about my conclusion.
