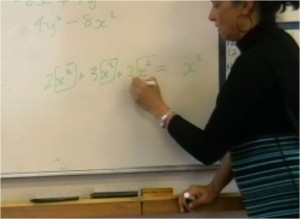We often provide a concrete example to clarify, so is it any wonder that confusion ensues when the task at hand may be purely abstract?
“But what is x?” The students need x to be something.
My students ask me, “Do you want an answer?”
“There are no numbers so I can’t give you an answer.”
I demonstrate specific examples of domains and ranges for my students because they arrive in my classroom with little or no knowledge of the uses and limitations of algebraic modelling.
My students accept the idea of an unknown and they are usually quite adept at solving simple problems like 7 + ∆ = 12. Most of my students are able to move to the idea 7 + n = 12, although, I recall during one diagnostic interview that when one student was asked to solve for n, given n + 5 = 18, he said 14 was the answer and explained that n was the 14th letter of the alphabet. When interviewing my students I have become aware that my students may not know that n can be a different number in subsequent problems. This realisation came to me when I noticed a student answering 13 for 5n = 30 because the value of n was 13 in the problem before it. Some my student explain that there is no solution possible because they are reading 5n as “fifty-something”, and not 5 “times” n.
How do we know unknowns are challenging for our students to learn?
We assess our student with an Solving Equations Diagnostic Test.
So what have we done to help our students understand unknowns?
We have changed the way we approach our teaching by:
- Showing students how algebra is everywhere in our lives
- Establishing what each student understands through assessment
- Promoting algebraic thinking with rich and meaningful contexts
- Demonstrating the benefits of rigour and correct use of vocabulary
- Building a “toolbox” of knowledge and skills that students can use appropriately and efficiently